[ENGN2211 Home]

Complex Power

In AC analysis it is very useful to use a complex generalization of power. The complex power   is defined by

$${\mathbf S} = {\mathbf V}_{rms} {\mathbf I}_{rms}^\ast ,$$

where the asterisk $^\ast$ denotes complex conjugate. This can be written

$${\mathbf S} = \vert {\mathbf V}_{rms} \vert \vert {\mathbf I}_{rms} \vert \angle \theta ,$$

where $\theta = \phi_v-\phi_i$, or as

S = P + j Q .

Here,

$P = {\rm Re}({\mathbf S})= \vert {\mathbf V}_{rms} \vert \vert {\mathbf I}_{rms} \vert \cos( \theta)$ is the real power. The units are watts.

$Q = {\rm Im}({\mathbf S})= \vert {\mathbf V}_{rms} \vert \vert {\mathbf I}_{rms} \vert \sin( \theta)$ is the reactive power. The units are vars (volt-amps reactive).  

    This is illustrated in Figure 40.

  

Figure 40: Complex power.

If the load has impedance Z then by Ohm's law,

V_rms = Z I_rms ,

and so

$${\mathbf S} = {\mathbf V}_{rms} {\mathbf I}_{rms}^\ast = {\mathbf Z} {\mathbf I}_{rms} {\mathbf I}_{rms}^\ast .$$

Thus

$${\mathbf S} = {\mathbf Z} \vert {\mathbf I}_{rms} \vert^2 .$$

Consequently,

$$P = {\rm Re} ( {\mathbf Z} ) \vert {\mathbf I}_{rms} \vert^2 ,$$

and

$$Q = {\rm Im} ( {\mathbf Z} ) \vert {\mathbf I}_{rms} \vert^2 .$$

Note that Z is complex, but $\vert {\mathbf I}_{rms} \vert^2$ is real.

Consider the following cases.

Case 1. Z = R (purely resistive load). Then

$$P = R \vert {\mathbf I}_{rms} \vert^2, \ \ Q=0 .$$

Case 2. ${\mathbf Z} = j\omega L$ (purely inductive load). Then

$$P = 0, \ \ Q = \omega L \vert {\mathbf I}_{rms} \vert^2 .$$

Case 3. ${\mathbf Z} = \frac{1}{j\omega C}$ (purely capacitive load). Then

$$P = 0, \ \ Q = \frac{-1}{\omega C} \vert {\mathbf I}_{rms} \vert^2 .$$

Case 4. ${\mathbf Z} = R + j\omega L + \frac{1}{j\omega C}$ (series RLC). Then

$$P = R \vert {\mathbf I}_{rms} \vert^2, \ \ Q = (\omega L - \frac{1}{\omega C}) \vert {\mathbf I}_{rms} \vert^2 .$$

The reactance depends on frequency, and so does the reactive power absorbed.

Note that Q=0 if $\omega L - \frac{1}{\omega C} = 0$, or

$$\omega = \frac{1}{\sqrt{LC}} .$$

In this case, $\theta =0$ and the power factor is 1.

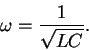
In general $\omega L - \frac{1}{\omega C} \neq 0$, and so $Q\neq 0$, and $-90^\circ \leq \theta \leq +90^\circ$.

If $-90^\circ \leq \theta \leq 0$, the load looks like RC (capacitive, $\omega L - \frac{1}{\omega C} < 0$) and the power factor is leading   (because current leads voltage).

If $0 \leq \theta \leq +90^\circ$ the load looks like RL (inductive, $\omega L - \frac{1}{\omega C} > 0$) and the power factor is lagging   (because current lags voltage).

This is illustrated in Figure 41.

  

Figure 41: Leading and lagging power factors.

[ENGN2211 Home]