RMS Values

V_rms is the root-mean-square value, given by

$\begin{displaymath}V_{rms} = \sqrt{ \frac{1}{T} \int_0^T [v(t)]^2 dt } . \end{displaymath}$

So if $v(t) = V_m \cos( \omega t + \phi_v)$ , we have

$\begin{displaymath}V_{rms} = \frac{V_m}{\sqrt{2}} . \end{displaymath}$

Similarly for current.

The formula for real AC power is

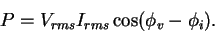$\begin{displaymath}P = V_{rms}I_{rms} \cos( \phi_v - \phi_i) . \end{displaymath}$

(51)

Real AC power is the product of the voltage and current RMS values times the cosine of the angle between the voltage and current phasors.

RMS phasors can be defined, e.g.

$\begin{displaymath}{\mathbf V}_{rms} = V_{rms} \angle \phi_v . \end{displaymath}$

ANU Engineering - ENGN2211