Here are just a few of McCormack's features:
The first chapter has 7x62 words and 7x7x44 letters, and the
second has 7x64 words and 7x7x47 letters.
The total number of words is 7x7x18 and the total number of
letters is 7x7x7x13.
Of this the verbs give 7x19 words and 7x144 letters,
the proper nouns 7x23 words and 7x144 letters, the common
nouns 7x18 words and 7x144 letters, the pronouns 7x8 words
and 7x36 letters, and the adverbs 7x4 words and 7x16 letters.
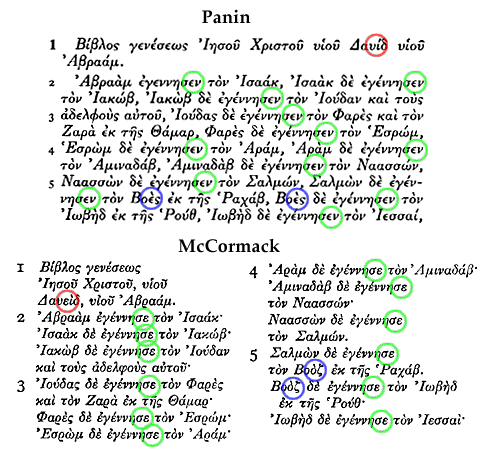
Considering the genealogy in the first 17 verses, a favourite topic of Panin, McCormack found that the proper names make 7x7x2 words and 7x7x12 letters.
And so on, for many pages.

In order to produce these patterns, they modified the text using the many variant readings that appear in old manuscripts. In addition to this deliberate cooking of the data, they presented some of the vast number of features of 7 that appear in any text by pure chance.
The only logical conclusion we can draw from this sorry episode is that neither author achieved anything beyond self-delusion.
Back to the Ivan Panin page
Creator: Brendan McKay,
bdm@cs.anu.edu.au.
Back to the Mathematical Miracles page